Problem 01: -14/29 just plug, crank, and pull.
Problem 02: 5/3
Conjugates are the red herring here.
(4√2 + √2)÷(4√2 - √2) = 5√2 ÷ 3√2 =5/3
Problem 03: 2
Draw a vertical line down the middle and the students will quickly see that the two halves are 2x2 and the red portion is 1+1 = 2
Problem 04: 112
Choose three consecutive integers: x-1, x, x+1
(x+1)³ - x³ = 666 + x³ - (x-1)³
x³ + 3x² + 3x +1 - x³ = 666 + x³ - x³ + 3x² - 3x + 1
Stuff cancels all over the place: 6x = 666; x = 111; Answer is 112
Problem 05: 1
Problem 06: 17
(x+2)² = x² + 8²
x² + 4x + 4 = 64 + x²
4x = 60; x=15; hypotenuse = 17. Even if you missed the Pythagorean triple.
Problem 07: 15
Problem 08: 36
Problem 09: 117
Problem 10: 59
Problem 11: 2
9x + 2(3x+2) = 243
32x + 18(3x) = 243
complete the square for U = 3x
u² + 18u + 81 = 243 + 81 = 324 = 18²
u + 9 = +- 18
3x = 9, -27 .... 2 is only real answer.
Problem 12: 13/14
Problem 13: 930
Problem 14: 140√2
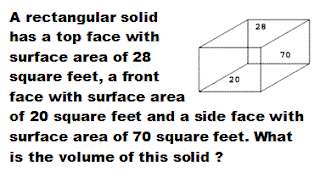
ab = 28; ac = 20; bc=70
a²b²c² = 28*20*70
abc = √(4*7*4*5*7*2*5) = 140√2
Problem 15: 6/31
In these simple dice rolling questions, I like to imagine the full sample space of 36 possibilities. We are told that 6 is not an option and that occurs 5 times, leaving 31 total.
11 12 13 14 15 16
21 22 23 24 25 26
31 32 33 34 35 36
41 42 43 44 45 46
51 52 53 54 55 56
61 62 63 64 65 66
Thus, P(7) = 6/31
Problem 16: 48
Problem 17: 62
Build a rectangle around the figure and subtract the four triangles.
10*10 - ½(4*7 + 3*7 + 3*3 + 6*3) = 62
Problem 18: 1/5
Problem 19: 6/55
Problem 20: 16
Problem 21: 10
Problem 22: 1/13
Problem 23: 8
Problem 24: 4013
Problem 25: 6
log144(3a * 2b) = c
144c = 3a * 2b
24c * 32c = 2b * 3a
b=4c and a=2c; answer 6
Problem 26: a=3, b=2/13
Problem 27: 1/6
Problem 28: 49
Problem 29: 5π/8
Problem 30: 300
Problem 31: 64/175
Problem 32: 18√3
ABC is a 30-60-90, with B=90o. AC is 12, split 3,3, and 6. If the altitude is BN, then AN=3 and BN=3√3, making the area = 18√3
Problem 33: 3
Problem 34: 339
4a+4b+4c=124, so a+b+c=31. Square it
a²+ab+ac+ba+b²+bc+ca+cb+c²=961
2ab + 2ac + 2bc = 622
a² + b² + c² = 339, which is also d²
Problem 35: 250
Assume first that p is odd. The list of integers is symmetric:
x-2, x-1, x, x+1, x+2; 5x = 57
In general, then px = 57, making p a power of 5.
p=5, x=56. 5 terms
p=25, x=55. 25 terms
p=125, x=54 = 625 terms.
p=625, x=53. Impossible because x was the "middle" number and we can't have 312 on either side.
Assume p is even.
x and x+1 are the middle two terms.
x-3, x-2, x-1, x, x+1, X+2, x+3, x+4 : 8 terms => 8x + 4
In general, p terms = px + .5p = p(x+1/2).
Since x is integer, we need a 2: q(2x+1) = 57.
5(2x+1)=57: x=56, means ten terms.
25(2x+1)= 57: x=1562, 50 terms
125(2x+1)=57: x=310, 250 terms
625(2x+1)=57: x=62, impossible for x to be the middle.
Problem 36: 46
logab + 10logba = 7
logab + 10/logab = 7
U + 10/U = 7
U² + 10 = 7U
U² - 7U + 10 = 0 ... (U-5)(U-2) = 0 .... logab = 2, 5
a5 = b has three candidates in range: 2,32; 3,243; 4;1024
since 45² = 2025, a² = b has 43 candidates in range. 2² = 4 through 44² = 1836
46 candidates.
Problem 37: 19
Problem 38: a=9 and b=11
Two equations:
386
a = 272
b
146
a=102
b
Starting with the second: 1a² + 4a + 6 = 1b² + 0b + 2
a² + 4a + 4 = b²
(a+2)² = b² gives us a+2 = b
Substitute into equation 1
3a² + 8a + 6 = 2b² + 7b + 2
3a² + 8a + 6 = 2(a+2)² + 7(a+2) + 2
expand
3a² + 8a + 6 = 2a² + 8a + 8 + 7a + 14 + 2
3a² + 8a + 6 = 2a² + 15a + 24
a2 - 7a - 18 = 0
(a+2)(a-9) gives us a = -2, 9
a = -2 is impossible so we're left with a=9 and thus b=11
back to
number 38.
Problem 39: 224x³
Simplify by canceling an x³ and then by substituting u = x³. Now for some long division. Yay!
u666 +2u663 +3u660 + u657 + ...
-----------------------
u3-1) u669 + u666 + u663 + u660 + ...
u669 - u666
-----------
2u666 + u663
2u666 -2u663
------------
3u663 + u660etc.
So each column adds one and projecting down the line gets you to a remainder of 224x³
Hang on. I messed that up. Must revisit.
u668 + u667 + u666 + u657 + ...
. -----------------------
u-1) u669 + 0 + 0 + u666 + 0 + 0 + u663 + u660 + ...
u669 - u668
-----------
u668 + 0
u668 - u667
------------
u667 + u666
u667 - u666
------------
2u666 + 0
When you are finished, you will have 224/u-1 but we can't leave it there. We removed a x³ from numerator and denominator so the last bit is really 224x³ / (x
6-x
3) so the remainder is really 224x³.
Good thing I wasn't taking the test for real.
Problem 40: 3/2
d² = x² +(2x)² +(5-x)² + (2x)² + (5-2x)² + (5-x)² + (5-2x)² + x²
Expand all that and cancel/clean up
sum of d² = 20x² - 30x + 50
min when d(sum)=0
40x - 60 = 0
x = 3/2
Kids get in trouble here because they want to differentiate the square root or get off the idea that the sum of the distances must be at a minimum.
Problem 41: 150
Cleaning up Jonathan's comment a bit:
The semiperimeter, S = (5+6+7)/2 = 9.
Heron's:
A = √(9*4*3*2) = √216 = 6√6.
To get Altitude (height):
Area = ½*5*h = 6√6 so h = 12/5√6
Use CA = 6 and h to find that CPh = 6/5.
Using h and Pythagoras:
q1 = (1/5)² + h²
q2 = (4/5)² + h²
q3 = (9/5)² + h²
q4 = (13/5)² + h²
Sum:
4(12/5*√6)² + (1+16+81+196)/25.
(4*144*6 + 1+16+81+196)/25 = 3750/25
= 150
Eric P said:
First find cos(C) by law of cosines
Cos(C)=[6²-7²-5²]/(-2*7*5)=19/35
q1² = 49 +1 -2(7)(1)(19/35)
q2² = 49 +4 -2(7)(2)(19/35)
q3² = 49 +9 -2(7)(3)(19/35)
q4² = 49 +16-2(7)(4)(19/35)
Lining it up this way and using some quick mental math tricks makes the addition simpler: SUM=4(49)+30-2(7)(10)(19/35)=150
I think I have to side with Eric on this one.
